Please note that Rice undergraduates enrolled in MATH 101, 102, 211, or 212 have access to free support for these courses through drop-in peer education offered in the O’Connor Building for Engineering Sciences each Sunday through Thursday from 7-10pm, with an additional session each Sunday from 2-5pm.
Welcome to the Department of Mathematics
As the current chair of the department, I join with my colleagues in welcoming you to the department. Located in Herman Brown Hall, the department comprises about 15 permanent faculty, 10 visiting faculty holding postdoctoral or instructorship positions and 30 graduate students. Read full message from the chair.
Brandon Levin Earn NSF CAREER Award
The award will support his work, "Models for Galois Deformations and Applications."
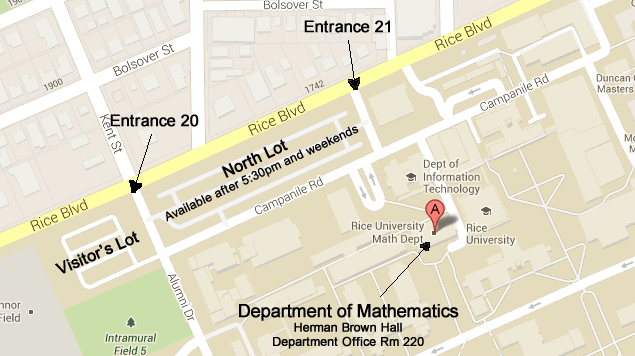
Contact Information
Tel (713) 348-4829
Mailing Address:
Rice University
Math Department -- MS 136
P.O. Box 1892
Houston, TX 77005-1892
Physical Address:
Rice University
Herman Brown Hall for Mathematical Sciences
6100 Main Street
Houston, TX 77005